In a previous log (https://hackaday.io/project/11225-a-new-high-accuracy-tilt-sensor/log/46957-theoretical-background-of-the-linear-fcdt) I showed that in case oftilt degrees, the volume of ferrofluid found in the (left) secondary coil is:And in case of tilt degrees, the volume of ferrofluid found in the (left) secondary coil is:In this log I'm going to show you where this formula comes from.

---

First, we need to slice the sensor at a point, and calculate the area of the ferrofluid at that point. This area should only depend on values we already know, such as the alfa tilt degree, the L coil length, the R radius, and the x variable. If got the area at that point, we can integrate it along x, and we get the volume of the ferrofluid.

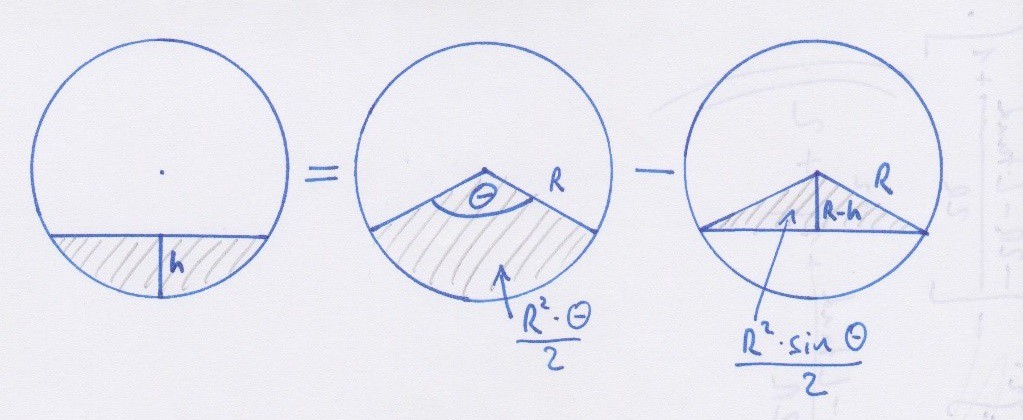According to the picture above, the area of the fluid is:

Next, we have to express theta with respect to R and h, since we only know the value of R (and we will know the value of h after the next step). We can do it using the simple angle-dependent cosine: 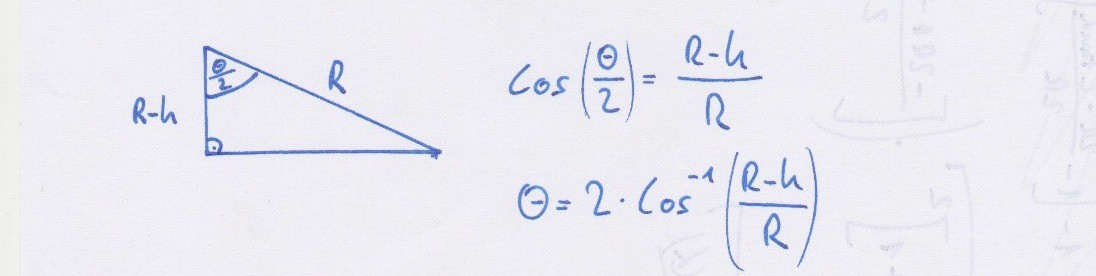With this, the formula of the area looks like this: Now there is only one variable we don't know: h. Let's determine it!

At a chosen x, h equals to:

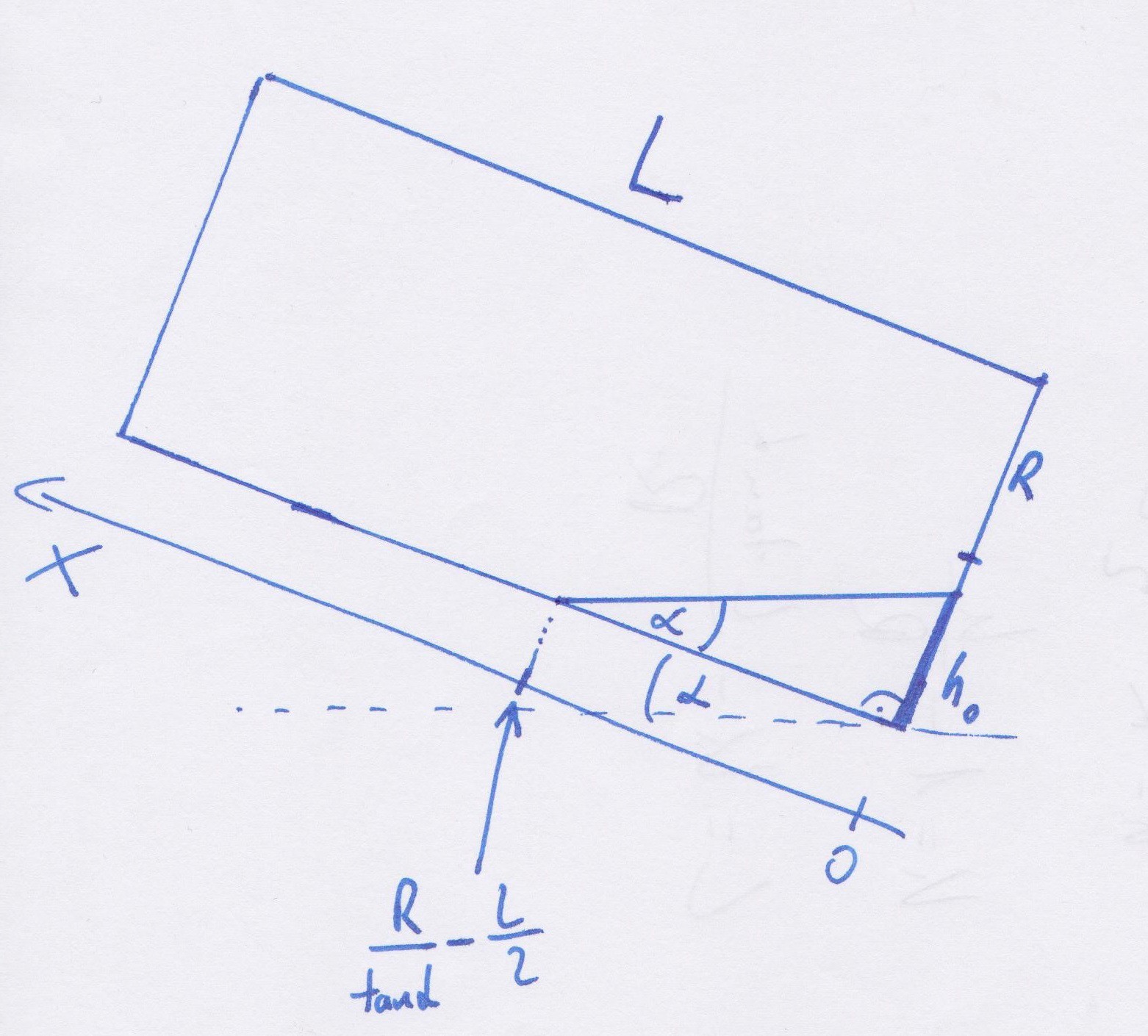
And from the picture above, h0 is:Substituting h0 to h's equation:
And that's it! The area of a slice looks like this:In the formula, the area depend on the R radius, the L coil length, the alfa tilt degree, and the x variable. Our last thing to do is to integrate it along x, and we get the volume of ferrofluid in a coil. But note that in cases of

we integrate from 0 to L, and in cases ofwe integrate from 0 to

---