Ideally we will collect data from the unit and calculate our cruise AoA, landing AoA (1.3 Vso) and stall speed Vso from empirical data. But to get started I looked into the theoretical AoA information for the PA-18 Supercub, our intended test bed.
Found The Incomplete Guide to Airfoil Usage to determine the airfoil in use on a PA-18, which is the USA 35B. Found NACA Report 233 on the airfoil as well as listing in Airfoil Tools
Reynold's number
Been a hot minute since I calculated Reynold's number, so asked to phone a friend. Need to vet this but here's a start.
Certainly! Let's calculate the Reynolds numbers for the given velocities:
- At 35 mph (15.65 m/s): Re=(1.225 kg/m3)⋅(15.65 m/s)⋅(1.6 m)(1.81×10−5 Pa\cdotps)Re=(1.81×10−5 Pa\cdotps)(1.225 kg/m3)⋅(15.65 m/s)⋅(1.6 m) Re≈1.76×106 Re≈1.76×10^6
- At 50 mph (22.35 m/s): Re=(1.225 kg/m3)⋅(22.35 m/s)⋅(1.6 m)(1.81×10−5 Pa\cdotps)Re=(1.81×10−5 Pa\cdotps)(1.225 kg/m3)⋅(22.35 m/s)⋅(1.6 m) Re≈2.51×106 Re≈2.51×10^6
- At 70 mph (31.29 m/s): Re=(1.225 kg/m3)⋅(31.29 m/s)⋅(1.6 m)(1.81×10−5 Pa\cdotps)Re=(1.81×10−5 Pa\cdotps)(1.225 kg/m3)⋅(31.29 m/s)⋅(1.6 m) Re≈3.51×106 Re≈3.51×10^
- At 110 mph (49.16 m/s): Re=(1.225 kg/m3)⋅(49.16 m/s)⋅(1.6 m)(1.81×10−5 Pa\cdotps)Re=(1.81×10−5 Pa\cdotps)(1.225 kg/m3)⋅(49.16 m/s)⋅(1.6 m) Re≈5.50×106 Re≈5.50×10^6
So something like 2,000,000 to 6,000,000
Airfoil Tools gives us the following plots for Re 500,000 and 1,000,000
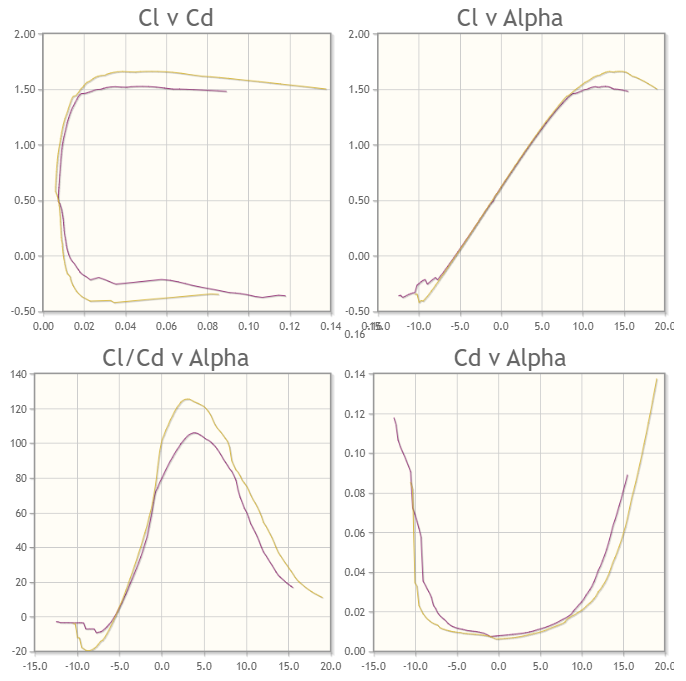
 tklenke
tklenke
Discussions
Become a Hackaday.io Member
Create an account to leave a comment. Already have an account? Log In.