[En svensk version finns i slutet av inlägget]

To choose the right size of motor

When it came to choosing the motor and inverter I started by selecting a maximum speed I would like to drive at continuously for 30 minutes, and a speed I would like to be able to drive indefinitely at. My initial thought was that I am willing to loose 1 Knot as maximum speed, which means a new max speed of 6 knots. And continuously I would like to not go slower than 4 knots. 

From my calculations, that means a motor that can deliver ~10kw for 30 minutes and 2kw continuously. However, since 30 minutes is a rather long time compared with how quickly an electric engine heats up, the continuous rating will have to be close to this. 

One more thing that I needed to know is at which RPM the engine shall be able to deliver this, and that is simply to look up the propeller rpm at the 10kW. Which, looking at the logged data, is around 850rpm. However, electric motors usually gett a high power output by spinning fast, and that is a problem since the torque goes down proportional to the rpm increase for a specific power output, since: power[w] = torque[Nm] * rotational speed[rad/s]. 

So the motor has to be able to deliver 10 kw at 850 rpm, and the torque delivered is then around 110 Nm, which is quite a lot. To compensate for this the diameter and pitch of the propeller could be decreased, or some type of gear reduction could be installed.

The things that I mainly considered was buying a kit or similar parts and using a toothed belt drive to increase the torque delivered by the engine. This seems like a popular DIY solution and I was about to jump on board that ship when I found a Tesla SDU (the front axel or FDU) on sale at Blocket (Swedish version of Craigslist/eBay) for a better price than the other solutions I had looked at. Before I decided to not board the usual DIY boat and sail by myself I tried to find some data on the motor and what it would mean for me. 

Tesla Small Drive Unit

This is what I found: 

Tesla Small Front Drive Unit Specifications.

Weight 90 kg (198 lbs) without driveshafts.

Max Speed 18,000 RPM

Transmission 9.34:1

Voltage Range 200-420 Volts DC

Max Current 650 Amps DC

Max Power 220 kW (300 Hp)

Max Torque 330 Nm (243 lb-ft)

Output Power (12 min.)90 kW (121 Hp)

Continuous Power 35 kW (47 Hp)

Max Regenerative Braking 90 kW (280 Amps)

Which clearly means that it has enough power. Apart from that I wanted to know how to control it as well as at which RPM it delivers the 35 kW.

For control, I ended up choosing to replace the logic board with one from https://openinverter.org/shop/ It is not the simplest solution since it requires opening up the inverter, but it was the cheapest and the way that would give me the most control which is why I chose to go with it. 

When it came to when the motor delivers 35 kW, I made a big assumption about there being no losses. 

At around 70 kph the Tesla comes into constant power delivery, which can be seen in the picture below, so the motor should be spinning slower than then to be able to run at the optimal slip.

Assuming standard 19-inch (0.4826m) wheels it means that they are spinning with

rpm. This means that the motor after the gearbox is spinning at 770x9.34=7187 rpm.

Since torque output is the main reason for heat at lower speeds and loads, therefore the max torque is more or less constant through the speed range. We can now calculate the continuous torque the motor can supply. 

Nm out of the gearbox.

434/9.34=46.5Nm from the motor.

Similarly, an estimate of the torque the motor can deliver at 18000 rpm can be calculated 

Which after the gearbox becomes: 18.6x9.34=173Nm.

From this, it seems that the gearbox is essential to get the torque needed. This however means that I need to get the differential welded or buy a kit with a shaft that locks it in place. As the gearbox only pumps oil for cooling when it is running in the forward direction it has to be mounted so it runs forward when the boat is being propelled forward, or it has to be modified.

I decided to go with the FDU/SDU and I also got to buy a Brusa nlg664 charger, which I hopefully will be able to use.

Svenska - Att välja rätt motor

När det gällde att välja motor och inverter började jag med att bestämma vilken maxfart jag vill kunna köra i 30 minuter, samt vilken fart jag vill kunna hålla kontinuerligt. Min första tanke var att jag kan leva med att tappa 1 knop i maxfart, vilket innebär en ny maxfart på 6 knop. Kontinuerligt vill jag dock inte köra långsammare än 4 knop.

Från mina beräkningar innebär det en motor som kan leverera cirka 10 kW i 30 minuter och 2 kW kontinuerligt. Men eftersom 30 minuter är ganska lång tid jämfört med hur snabbt en elmotor värms upp, måste den kontinuerliga effekten ligga ganska nära detta.

En annan sak jag behövde veta var vid vilket varvtal motorn ska kunna leverera detta. Det är enkelt att ta reda på genom att kolla propellerns varvtal vid 10 kW. Enligt loggdata ligger det runt 850 rpm. Problemet är dock att elmotorer vanligtvis levererar hög effekt genom att snurra snabbare, och då sjunker vridmomentet proportionellt med varvtalsökningen, eftersom: effekt[w] = vridmoment[Nm] * varvtal[rad/s].

Motorn måste alltså kunna leverera 10 kW vid 850 rpm, och vridmomentet är då cirka 110 Nm – vilket är ganska mycket. För att kompensera för detta kan man minska propellerns diameter och/eller stigning, eller installera någon form av växellåda.

Min första idé var att köpa ett kit eller liknande delar och använda en kuggrem för att öka vridmomentet som motorn levererar. Det verkar vara en populär lösning bland DIY-byggare, och jag var redo att hoppa på det tåget – eller snarare kliva ombord på den båten – när jag hittade en Tesla SDU (motorn för den främre axeln) på Blocket för ett bättre pris än andra lösningar jag tittat på. Innan jag beslutade mig för att inte borda den vanliga DIY-båten, försökte jag hitta lite data på motorn för att förstå vad det skulle innebära för mig.

Specifikationer för Tesla Small Front Drive Unit (SDU):

Vikt: 90 kg utan drivaxlar

Maxvarvtal: 18 000 rpm

Utväxling: 9,34:1

Spänningsområde: 200–420 V DC

Maxström: 650 A DC

Maxeffekt: 220 kW (300 hk)

Maxvridmoment: 330 Nm

Kontinuerlig effekt: 35 kW (47 hk)

Max regenerativ bromsning: 90 kW (280 A)

Det är tydligt att den har tillräckligt med effekt. Däremot ville jag veta hur den ska styras samt vid vilket varvtal den levererar 35 kW.

För styrningen valde jag att ersätta logikkortet med ett från https://openinverter.org/shop/. Det är inte den enklaste lösningen eftersom man måste öppna upp själva invertern (motorcontrollern), men det var den billigaste och gav mig mest kontroll – så det kändes rätt.

När det gäller vid vilket varvtal motorn levererar 35 kW gjorde jag några antaganden om att det inte skulle finnas några förluster.

Vid runt 70 km/h går Teslan över till konstant effektleverans, vilket man kan se i bilden nedan, så motorn borde snurra långsammare än detta för att kunna köras optimalt. 

Antar man Teslas standardhjul på 19 tum (0,4826 m) snurrar hjulen då med:

RPM. Det innebär att motorn innan växellådan snurrar med: 770x9.34=7187 rpm.

Eftersom vridmomentet är den största källan till värme vid lägre varvtal och belastningar, och maxmomentet är ganska konstant över hastighetsintervallet, kan vi nu räkna ut det kontinuerliga vridmomentet som motorn kan leverera:

Nm, vilket innan växellådan blir, 434/9.34=46.5 Nm från motorn.

På samma sätt kan man uppskatta vridmomentet som motorn kan leverera vid 18 000 rpm:

Nm, vilket efter växellådan blir: 18.6x9.34=173Nm

Det är alltså uppenbart att växellådan är avgörande för att uppnå det vridmoment som behövs. Men det betyder också att jag måste svetsa differentialen eller köpa ett kit med en axel som låser den. Eftersom växellådan endast pumpar olja för kylning när den körs framåt, måste den antingen monteras så axeln snurrar frammåt när båten går frammåt eller så behövs den modifieras.

Jag bestämde mig för att satsa på SDU:n och köpte dessutom en Brusa NLG664-laddare, som jag hoppas kunna använda.

[En svensk version finns i slutet av inlägget]

Background 

Two years ago I bought a boat with the hope that someday be able to electrify it. It is a displacement boat of a type called snipa or snäcka which typically has low drag at low speeds. For that reason I chose this boat type, the boat is a bit bigger than what I planned for, and I think that is the theme for most of this build. It is a Sandvik 25 and currently, it has a Volvo Penta MD3B as the engine. 

It has a displacement of 2400kg according to the old datasheet. But it is probably more with the equipment that has been added over the years. Before starting the conversion we exchanged the rubber gasket on all windows to keep the rain on the outside of the boat since that was a bit of a problem before. Because it does feel quite important to keep the water outside of the boat before installing a high-voltage battery in it. We also repainted the bottom as it was for lakes and not for the sea.

Initial testing

At the end of the first summer with the boat, we went out and made a small test logging the rpm of the motor, GPS speed as well as speed through water (not used). During the test we drove back and forth with one rpm setting before increasing it to 100rpm, writing down the speed after it had stabilized after each turn. After a total of 30 turns, we were done with the test, and the average results of the data look like this: 

My idea is to use this data to estimate the maximum power used to propel the boat at different speeds. I say maximum because it assumes that the engine can produce the power it could when it is brand new, which it most probably can not do. As well as there are other losses on the way to the propeller, which also should make the estimated power a bit higher.

A simple generic equation for static thrust can be found in this paper, which describes the relationship between the rpm and thrust using this equation: 

Where T_s is the static thrust produced (in newtons), K_t is the thrust coefficient, ρ is the air density (in kg/m^3), ω is the propeller speed (in rad/s), and D is the diameter of the propeller (in m). I have removed some constants and changed units since K_t will just be scaled accordingly.

It only holds for stationary propellers, so let's hope that the propeller moves somewhat slowly enough through the water. And that there is not too much growth on it making the equation break even more. But this should only make the estimated power used to propel the boat higher, resulting in a further range after the actual conversation.

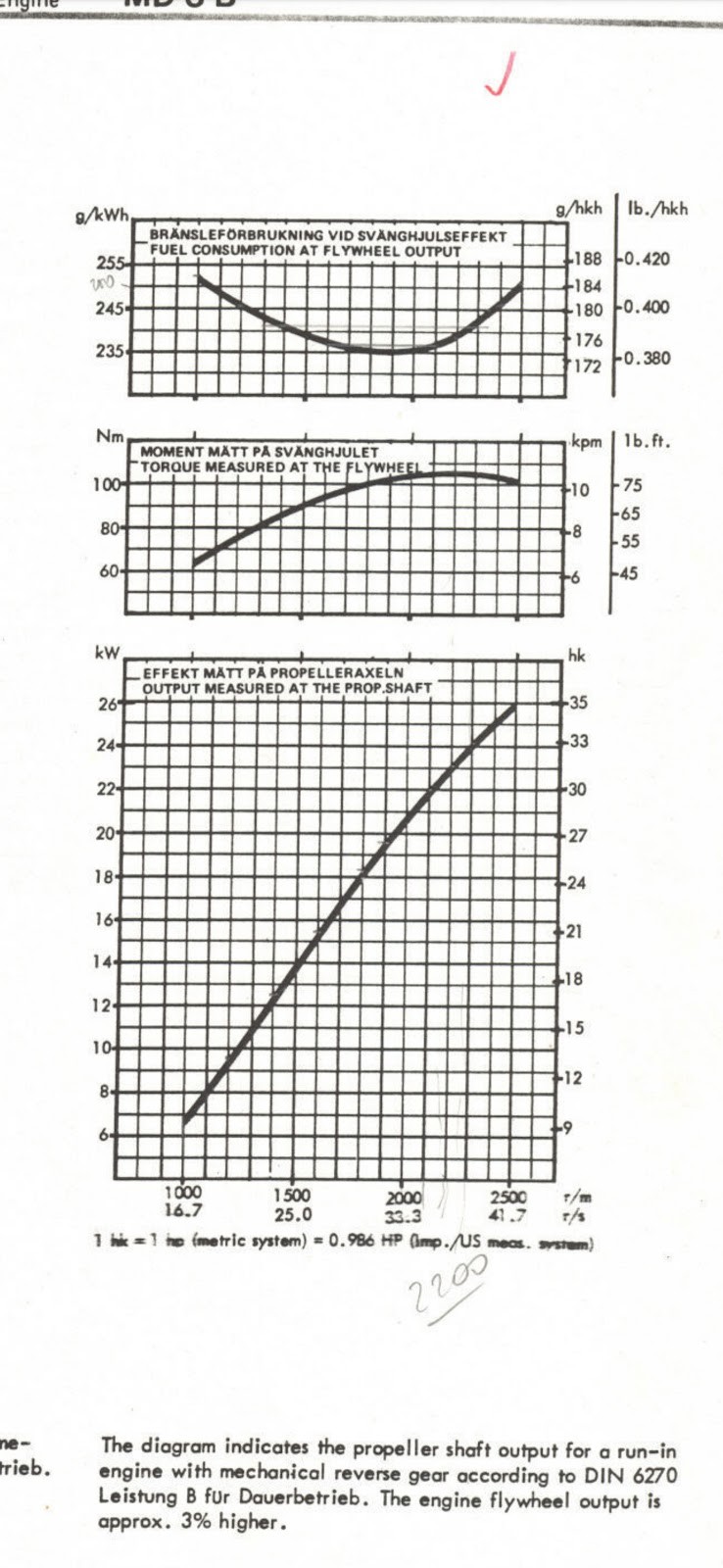
First, we find the power produced by the engine at the specific rpm. In the datasheet for the engine, such a point can be found for full throttle.

Then the rpm of the engine needs to be converted to the propeller speed, which has a reduction of 1.91 and then I also convert it to rad/s.

Since the speed of the boat, Vmax (in m/s), and power produced by the vessel, Pmax (in kW), is known at the max rotational speed of the propeller (in rad/s). We can solve for the thrust produced by the propeller: 

Fmax can then be plugged into our thrust equation, where it is called T_s, which we then can solve for the constant. This constant replaces all the other constants since they can be multiplied together. Resulting in this equation:

This new constant, K, can now be used to calculate the thrust produced at a certain rpm of the vessel, and since the velocity is already known the power used can be estimated. 

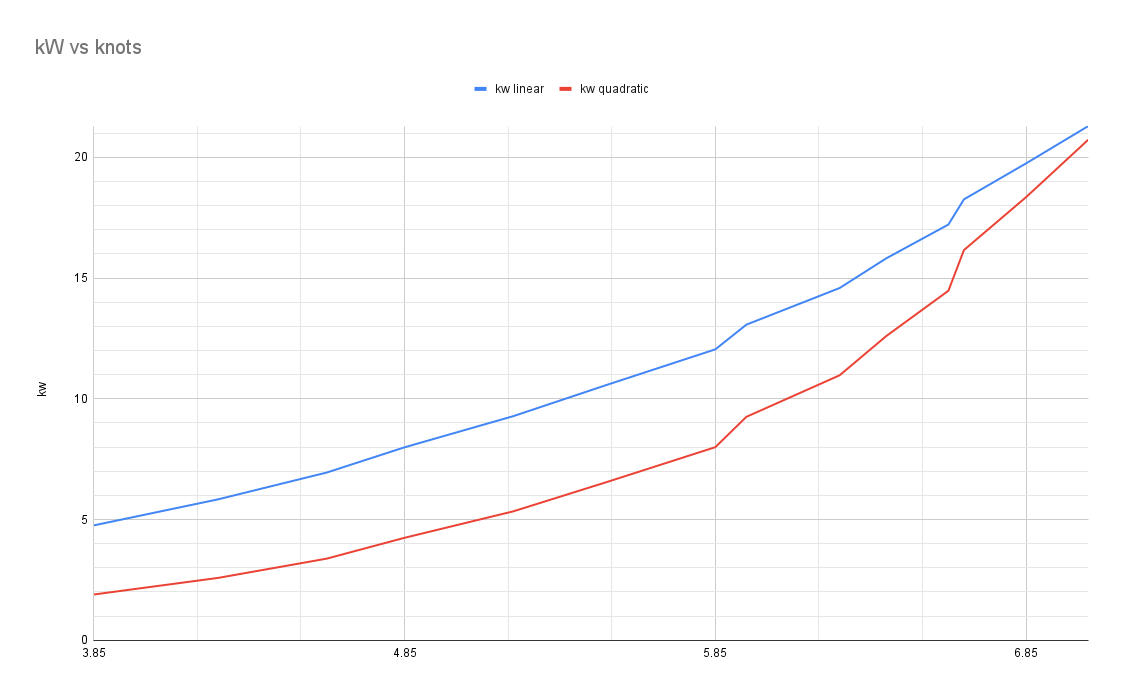
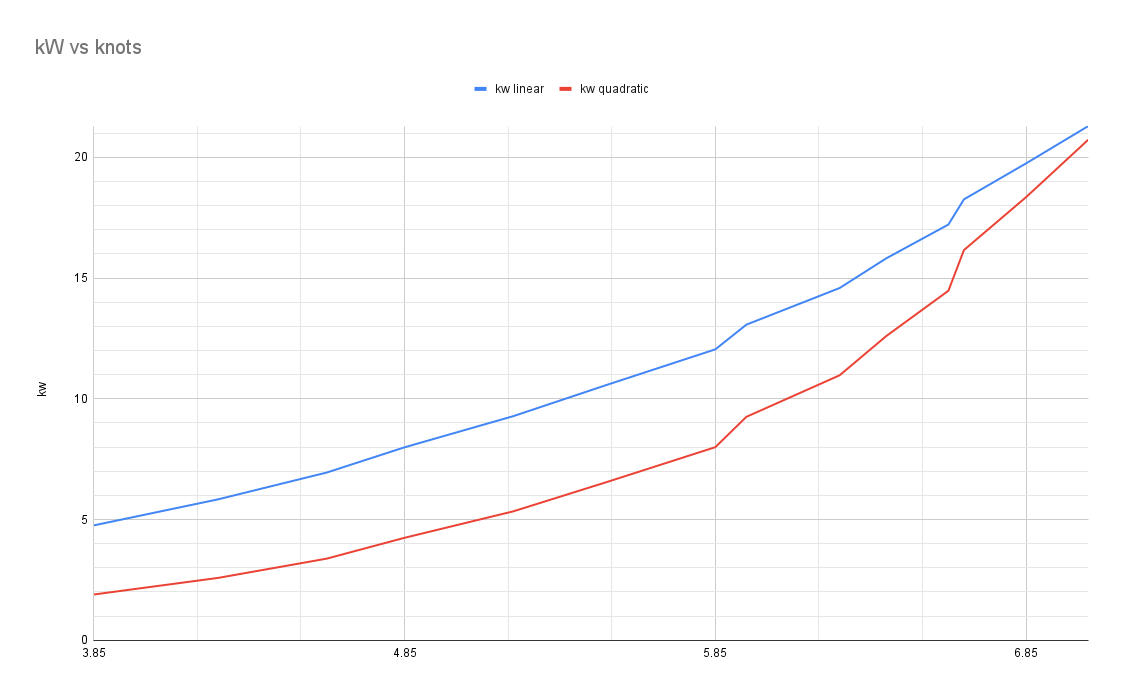
I did also do the calculations with a linear version of the formula (removing the square), to make a worse estimate at lower speeds. The electric motor will also have some inefficiency which will mean that more power will be pulled from the battery than is required to propel it forward. The results from both can be seen below:

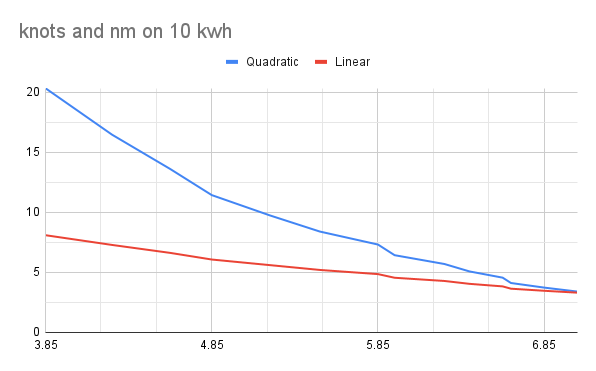
I have also done calculations on the range with a 10 kWh battery, which can be seen here (just divided the kilowatt-hours by kilowatt at a certain speed and multiplied it by the speed in knots): 

At the end of the post, the raw data can be found.

Svenska - Bakgrund 

För två år sedan köpte jag en båt med förhoppningen att någon dag kunna elektrifiera den. Det är en snipa, vilken vanligtvis har lågt motstånd vid låga hastigheter. Vilket är varför jag har valt den här båttypen, båten är lite större än vad jag planerade för, och jag tror att det är temat för det mesta av denna konverteringen. Det är en Sandvik 25 och för närvarande har den en Volvo Penta MD3B som motor. 

Den har en deplacement på 2400 kg enligt det gamla databladet. Men det är förmodligen mer med utrustningen som har lagts till under åren. Innan vi började konverteringen bytte vi ut gummilisten på alla fönster för att hålla regnet utanför båten eftersom det var lite av ett problem innan. Det känns ganska viktigt att hålla vattnet utanför båten innan man installerar ett högspänningsbatteri i den. Vi målade också om botten eftersom den var avsedd för Vänern och inte för västkusten.

Inledande testning

I slutet av den första sommaren med båten åkte vi ut och gjorde ett litet test där vi loggade motorns varvtal, GPS-hastighet samt hastighet genom vatten (används ej). Under testet körde vi fram och tillbaka vid ett visst varvtal innan vi ökade den med 100 RPM. Vid varje vända så skrev ner hastigheten efter att den hade stabiliserat sig. Efter totalt 30 rundor var vi klara med testet, och resultaten av datan ser ut så här: 

Iden jag har är att använda datan för att uppskatta den maximala effekt som används för att driva båten vid olika hastigheter. Jag tror att det är max eftersom min ide antar att motorn kan producera den effekt den kunde när den är helt ny, vilket den troligen inte kan göra. Iden antar även att all effekt som motorn producerar driver båten frammåt, detta är inte heller sant och de förlusterna som finns på vägen fram till propellern kommer också innebära att det beräknade kommer vara högre än verkligen.

En enkel ekvation för att beskriva förhållandet mellan varvtal och statisk dragkraft från en propeller finns i den här rapporten, och innehåller följande ekvation: 

Där T_s är den producerade kraften (i newton), K_t är dragkraftkoefficienten, ρ är luftens densitet (i kg/m^3), ω är propellerns hastighet (i rad/s) och D är propellerns diameter (i m). Jag har bytt enheter och plockat bort konstanter, men det kommer endast skala K_t.

Ekvationen gäller dock bara för stationära propellrar i öppet vatten, så jag får hoppas att min propeller rör sig tillräckligt långsamt och att det inte finns för mycket påväxt på den som gör att ekvationen fungerar ännu sämre. Men om det är fel så bör det bara göra den uppskattade effekten som används av motorn högre, vilket resulterar i ett längre sträcka som båten kan köra efter den faktiska konverteringen.

Först hittar vi den effekt som produceras av motorn vid det specifika varvtalet. I databladet för motorn kan man hitta en sån punkt vid full gas.

Sedan behöver motorns varvtal konverteras till propellerhastighet, bakslaget har en reduktion på 1,91 och sedan konverterar jag det också till rad/s.

Eftersom båtens hastighet, Vmax (i m/s), och effekten som produceras av motorn, Pmax (i kW), är känd vid den maximala rotationshastigheten för propellern (i rad/s). Kan vi lösa för den dragkraft som produceras av propellern: 

Fmax kan sedan sättas in i vår dragkraftsekvation, som vi sedan kan lösa för konstanten. Denna konstant ersätter alla andra konstanter eftersom de kan multipliceras tillsammans. Vilket resulterar i denna ekvation:

Denna nya konstant, K, kan nu användas för att beräkna den dragkraft som produceras vid ett visst varvtal på båten, och eftersom hastigheten redan är känd kan den användas för att uppskatta den använda effekten. Detta kan ses nedan:

Jag gjorde också samma beräkningar med en linjär version av ekvationen (tog bort kvaderaten) för att få ett ännu värre estimat. Den elektriska motorn kommer också att ha viss ineffektivitet vilket innebär att mer effekt kommer att dras från batteriet. Resultaten kan ni se här:

Jag har också gjort beräkningar av räckvidden med ett 10 kWh-batteri, vilket kan ses här (jag delade bara kilowattimmar med kilowatt vid en viss hastighet och multiplicerade med hastigheten i knop): 

Här under finns datan som jag har samlat ihop.

Data

Below you can find the data that we have collected and the calculations that I have done:

Nedan finns datan vi har samplat ihop och beräkningarna jag har gjort:

Quadratic:

knots	RPM (propeller)	force [n]	speed [m/s]	power [kw]	rad/s	Torque [nm]
3.85	471.2041885	956.7730036	1.980452675	1.894843654	49.34438723	38.40038879
4.25	523.5602094	1181.201239	2.186213992	2.582358676	54.82709692	47.10004397
4.6	575.9162304	1429.253499	2.366255144	3.381978445	60.30980661	56.07675823
4.85	628.2722513	1700.929784	2.494855967	4.243574822	65.79251631	64.49935433
5.2	680.6282723	1996.230094	2.674897119	5.339710128	71.275226	74.91677582
5.5	732.9842932	2315.154428	2.829218107	6.550076829	76.75793569	85.33419731
5.85	785.3403141	2657.702788	3.009259259	7.997716722	82.24064538	97.24773785
5.95	837.6963351	3023.875172	3.060699588	9.255173494	87.72335507	105.5040985
6.25	890.052356	3413.671581	3.215020576	10.97502437	93.20606477	117.7501099
6.4	942.408377	3827.092014	3.29218107	12.59947988	98.68877446	127.6688251
6.6	994.7643979	4264.136473	3.395061728	14.47700654	104.1714842	138.9728356
6.65	1047.120419	4724.804956	3.420781893	16.16252724	109.6541938	147.3954317
6.85	1099.47644	5209.097464	3.523662551	18.35510166	115.1369035	159.4197959
7.05	1151.832461	5717.013997	3.62654321	20.73299829	120.6196132	171.8874546

Linear:

knots	RPM (propeller)	force [n]	speed [m/s]	power [kw]	rad/s	Torque [nm]
3.85	471.2041885	2402.56332	1.980452675	4.758162954	49.34438723	96.42764296
4.25	523.5602094	2669.5148	2.186213992	5.836130607	54.82709692	106.4460994
4.6	575.9162304	2936.46628	2.366255144	6.948428441	60.30980661	115.2122487
4.85	628.2722513	3203.41776	2.494855967	7.992065914	65.79251631	121.473784
5.2	680.6282723	3470.36924	2.674897119	9.282880684	71.275226	130.2399334
5.5	732.9842932	3737.32072	2.829218107	10.57369545	76.75793569	137.7537757
5.85	785.3403141	4004.2722	3.009259259	12.04989319	82.24064538	146.519925
5.95	837.6963351	4271.22368	3.060699588	13.07293256	87.72335507	149.0245391
6.25	890.052356	4538.17516	3.215020576	14.59032652	93.20606477	156.5383814
6.4	942.408377	4805.12664	3.29218107	15.81934696	98.68877446	160.2953026
6.6	994.7643979	5072.07812	3.395061728	17.22001831	104.1714842	165.3045308
6.65	1047.120419	5339.0296	3.420781893	18.26365578	109.6541938	166.5568378
6.85	1099.47644	5605.98108	3.523662551	19.7535856	115.1369035	171.566066
7.05	1151.832461	5872.93256	3.62654321	21.2984437	120.6196132	176.5752943

Time/Distance:

knots	time on 10kwh Quadratic	nm on 10 kwh Quadratic	time on 10kwh Linear	nm on 10 kwh Linear
3.85	5.27748027	20.31829904	2.101651435	8.091358025
4.25	3.872428758	16.45782222	1.713464052	7.282222222
4.6	2.956849124	13.60150597	1.439174352	6.62020202
4.85	2.356503755	11.42904321	1.251240932	6.068518519
5.2	1.872760835	9.738356345	1.077251808	5.601709402
5.5	1.52669965	8.396848073	0.9457431457	5.201587302
5.85	1.250356864	7.314587654	0.8298828743	4.854814815
5.95	1.080476774	6.428836806	0.7649393091	4.551388889
6.25	0.9111597078	5.694748174	0.6853856209	4.283660131
6.4	0.7936835562	5.07957476	0.6321373457	4.045679012
6.6	0.690750534	4.558953524	0.5807194755	3.832748538
6.65	0.6187151211	4.114455556	0.5475355054	3.641111111
6.85	0.5448076608	3.731932477	0.506237207	3.467724868
7.05	0.4823229067	3.400376492	0.4695178738	3.31010101